Algebra 1 Unit 1 Interactive Notebooks [Revised]: Solving Equations and Inequalities

This year I’ve committed to posting each unit of both my Algebra 1 and Algebra 2 INBs.

My district is moving to a standards based curriculum, and has identified priority standards for every course. These are the standards we are required to address and assess our students over, so they pretty much form our units.

I normally teach year-long courses (47 minute classes per day). However, due to a lot of district specific things involving SPED students, a new course introduction, and graduation requirements, this year I taught a block course (94 minute classes per day) of Foundations of Algebra first semester, which covered skill gaps students would need to find success in Algebra 1. This semester, I am teaching (most of) those same students Algebra 1 on a block schedule. This means I get to start Algebra 1 from the beginning in the same year and revise my activities and INB pages!

I took a poll on twitter and many of you said you would like me to still include pages that I didn’t change, so that’s what I’m doing, but I’m not going to write extensively about those pages.

You can find my Algebra 1 (year long class) INB posts here:

Unit 1 | Unit 2 | Unit 3

And my Algebra 2 INB posts here:

Unit 1 | Unit 2 | Unit 3 | Unit 4

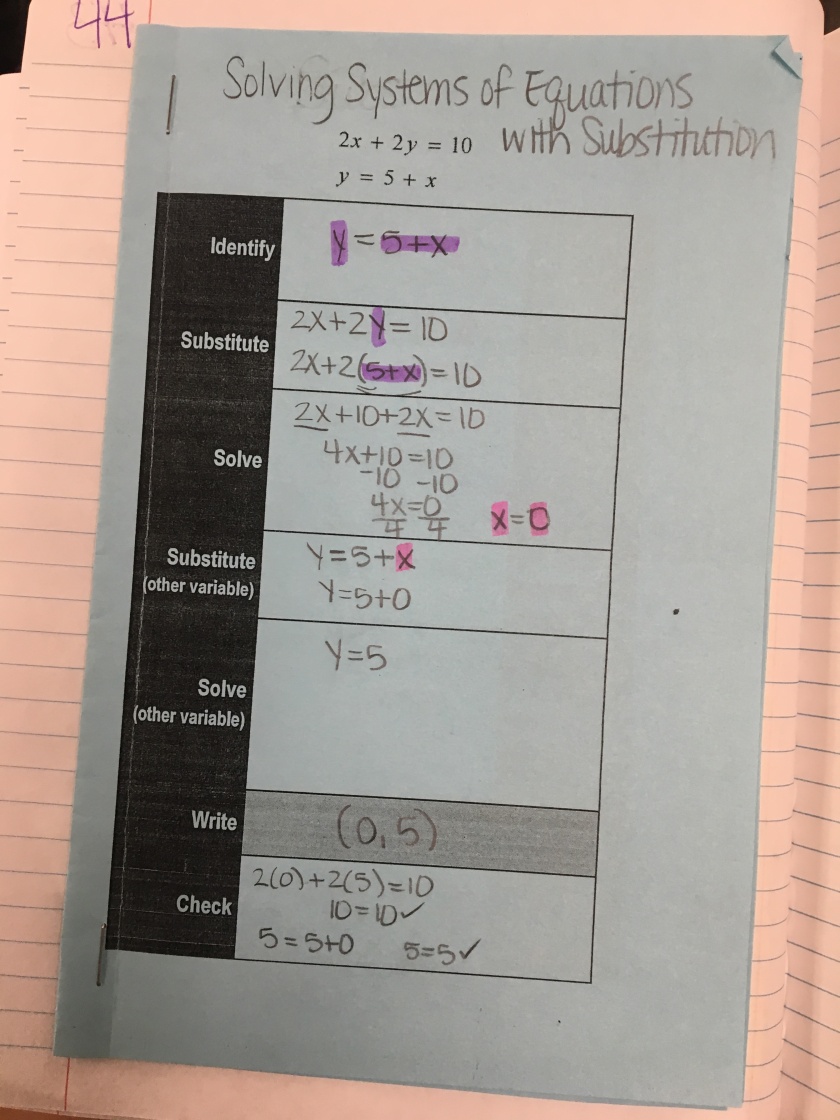
The first standard we prioritize in Algebra 1 is A.REI.B.3: Solve linear equations and inequalities in one variable, including equations with coefficients represented by letters.

You can read more about my INB index pages in other posts. I have added to the back side of them a proficiency tracking log that I am not sure how I feel about yet, so I will save discussing that for my next unit’s post!

Skill 1: I can evaluate expressions and check possible solutions to equations.

To quote myself from the first time I taught this unit this year…

Next year when I teach this, I want to add another skill to the beginning that is just evaluating equations/expressions for given values of a variable. I meant to do this, and then I just forgot, but one of the things my students have been struggling with most is actually inputting their solutions correctly into a calculator to check them! Then they end up thinking their solution is incorrect, when really it’s their evaluation that’s incorrect…

So, here that skill is! I do think it made my students more comfortable with what their goal was in solving equations and what their solutions meant. I stole the parenthetical promise from Sarah Carter, and we immediately looked at why it matters. We don’t deal with exponents much in our first three units, so I guess I won’t really see the effect this has until next quarter, but I think it was worth talking about right at the beginning. We also discussed that calculators don’t understand that negatives and subtracting are the same idea.

Skill 2: I can solve one and two step equations

The only changes I made from my original pages here were to include the actual property names in the “legal math moves” section, to make the definition boxes bigger on the front (the picture here is the original and you can see how cramped it was), and to show students both the traditional solving algorithm AND a do/undo list/chart for solving. I think all of these changes were good, but especially the do/undo solution method. This REALLY helped when we got to literal equations, but I’ll talk about that later.

All of these students came from the Foundations of Algebra class I mentioned, and that course ENDS with an introduction to simplifying expressions and solving equations. So all of these students had seen equations like this quite recently and we were able to go quickly through these skills. Nothing was brand new to them until literal equations, but repeating this gave a lot of students the chance to master this that were still confused before.

Skill 3: I can solve equations with like terms and distribution.

I didn’t change the pages for this skill at all! You can read a more thorough description in this post.

Skill 4: I can solve equations with variables on both sides.

I added a page here on possible results of solving an equation. The first time I taught this this year, we addressed these possibilities within the examples but I felt like students did not get a strong understanding that they were NOT solving the equations wrong when they ended up with 2=4. I think adding this page helped to combat that. I also adjusted the organizer for the examples to have less steps than before – I included moving the variables to one side in the “solve” step instead of separating it. Looking back, I think I might need to put that back in as a separate step because this was a stuck point for a lot of students, but I really don’t want to lock them in to ALWAYS moving variables first. I will have to think about this one more.

Skill 5: I can solve literal equations (equations with >1 variable).

I honestly feel like the do/undo chart was a mini miracle for my students. They were SO MUCH MORE SUCCESSFUL in solving literal equations than I have ever had students be the first time around. The only time it doesn’t work is if you have two instances of the variable, and then you have to simplify first before you can make the chart.

We did the same literal equations scavenger hunt that I’ve been doing for a few years now, where you have to get all of the equations into slope intercept form (except the students don’t know that’s what it’s called yet), and I had zero panic. ZERO PANIC. It was amazing. Obviously, I’ll have to teach it this way a few more times, but I think this was a breakthrough for me as a teacher.

I’ll need to address the multiple instances of the variable you’re solving for in my next version of these notes.

Skill 6: I can solve one and two step inequalities.

Another set of pages I did not alter – I like these and I think they’re effective. My students did continuously forget when to flip the inequality symbol even when we talked about it extensively. We had a discussion about how when you read your solution you should be talking ABOUT THE VARIABLE, for example “x is greater than 1” instead of “1 is less than x”, which is talking about 1 and not x. That helped with that understanding, so I may include that in my notes next time. I don’t know how else to get them to look out for multiplying and dividing by a negative number – we illustrated why it needs to happen, we listed it before we started every practice, and still the majority of them forgot to reverse the inequality symbol. Suggestions?

Skill 6: I can solve inequalities with multiple steps.

 

 

 

All of the Unit 1 A.REI.3 pages pictured in this post can be found here. Most of them can be downloaded in PDF or Publisher (editable) form. Within that link is a folder to the pages I used the first time around this year that I didn’t use this time around, if you are interested in those.

Algebra 1 Unit 3 Interactive Notebooks: Systems of Equations

This year I’ve committed to posting each unit of both my Algebra 1 and Algebra 2 INBs.

My district is moving to a standards based curriculum, and has identified priority standards for every course. These are the standards we are required to address and assess our students over, so they pretty much form our units.

Algebra 2 INB Posts:

Unit 1 | Unit 2 | Unit 3 | Unit 4

Other Algebra 1 INB Posts:

Unit 1 | Unit 2

 

The third standard we cover in Algebra 1 is A.REI.6:

Solve systems of linear equations exactly and approximately (e.g., with graphs), focusing on pairs of linear equations in two variables.

On the index page, you can see that I divided this standard up into 6 skills. I pretty much like the way I organized this, except for the way I presented the elimination method, which I’ll address more when we get to those pages.

Skill 1: I can determine if a given point is a solution to a system of equations

We started out with a definition of what a system of equations is. I think this made students comfortable moving forward with understanding what they were looking at and what was going on. We also did the Desmos activity Playing Catch-Up before we discussed this to kind of expose them to the concepts. I wish we had had time to revisit this activity at the end of the unit, but with a day cancelled due to cold and very shortened days before winter break, we ended up not having time to do that. I would have loved to see how much further my students could have gotten on the tasks after studying systems for over a month!

This is a skill that I previously have neglected to address separately – checking your solution. I think this is the best thing that I did this entire unit. My students got really comfortable checking points to see if they were solutions and most of them continued to check the solutions they found throughout the entire unit. This made giving them help so much easier because they came to me as soon as their solution didn’t check out instead of continuing on through an assignment with misconceptions! All of my students ended up settling on the parentheses method instead of storing values, but our district explicitly tells us to teach them how to store values in the TI-83 Plus, so I will keep presenting it as an option.

After we took the notes for this section we played Roll-A-Solution to practice, which the students loved. I wrote a blog post on this game.

Skill 2: I can graph a system of equations to find the solution

I like the way I set up the process for these notes, but I think maybe I went overboard on the number of examples for them to include in their notebooks (there are 6 in this packet). This took us forever and many of my students gave up halfway through so they only have half of these examples anyways. I think that in the future I will have them graph an example with one solution, an example with no solutions, and an example with infinitely many solutions, with one of the examples having the equations not already solved for y.

I really liked introducing the three possible outcomes for a system of equations right away, and previewing how students would know which one they were encountering. I strongly dislike presenting systems with no solutions and systems with infinitely many solutions as “special cases” because students end up thinking that they have to solve them differently. This year I integrated these types from the beginning as possibilities and it went really well.

Skill 3: I can solve a system of equations when given the value of one variable

I made the decision when planning this unit out this year to include this intermediate step to the substitution method of solving systems – cases where one variable’s value is just given to you. This then becomes really a case of just solving one equation, but students still go through the motion of replacing one variable with something to start with. I suspected that this would help students really grasp the idea of substituting without all of the complexity – and I think this was true! My students ended up being the strongest in substitution that they’ve been through my 5 times teaching this unit and I think this step was a large part of it.

I kicked this off with a notice/wonder, which I then had them attach to these notes so that they could remember this was a certain type of system. I also utilized highlighters extensively here – throughout the notes we highlighted values that were equivalent and then discussed that you could exchange any highlighted value for any other highlighted value. I think this was really helpful for my students as they got started using these methods, and most of them abandoned the highlighters as they got more comfortable.

Skill 4: I can solve a system of equations using substitution.

Here we use the same process as in the previous skill, except now you need to substitute and solve again to find the value of the other variable, since we don’t already know it for every system of equations. This went really smoothly after practicing the previous skill and I’m really happy with my students’ proficiency using this method! I did forget to type the name of the method at the top of these notes though. Notice the two highlighter colors – everything in one color is equivalent to x, and everything in the other color is equivalent to y!

Skill 5: I can solve a system of equations using elimination

This skill did not go as smoothly. I really wanted to go over the concepts you see on the front of the foldable, which are the concepts that allow us to use the elimination method. However, I now don’t think it was really necessary to include these written in their notes – I think that in the future I will go over this exact page as a class, but only have examples of the actual elimination method in their notes.

I also need to adjust the way I instructed regarding this method – my students ended up being super confused about how to end up with the opposite variables if they weren’t already opposite and we needed to have way more notes and practice with just that part, whereas the rest of the process was pretty intuitive to them once we got to that point. I think that I might design a new foldable with one page of practice just on “get either x terms or y terms to be opposites using multiplication” and then have the rest be examples, or split this into two separate pages, one that is just practicing the actual elimination and solving with a pair of variables already being opposites, and one where they have to create the opposites using multiplication. I also plan on adjusting the steps a little bit and the sizes of the respective boxes.

Skill 6: I can write and solve a system of equations to represent a situation

After we had learned the three solution methods that get covered in Algebra 1, we used this foldable to discuss the “most efficient method”. I emphasized that this was not the method they had to use, but it was the method that would take the least amount of work for that specific system, which is something my students are usually interested in! We did practice identifying that method together, and then they did a practice assignment where they could choose whatever method they wanted to solve the system. Most of them ended up using the most efficient methods, but there were a few who had a strong preference toward either substitution or elimination, and a few who just really did not want to graph any of them.

Then we also discussed writing systems of equations from a situation. This skill is not explicitly listed in the standard, so it isn’t assessed on any of our district assessments – they are sometimes given a situation AND given the system, so that they have to interpret their solution in a context, but they aren’t required to write the system themselves. I figured this would be good practice on writing equations in general though, which was also on their final assessment from our previous unit, so we took a look at this skill anyways. In hindsight, I wish that I had chosen at least one situation that wasn’t about money! Otherwise my students mostly showed that their understanding of how to write an equation has improved since our last unit. The main struggle was separating what pieces of information needed to go in which equation, especially when the situation said “the total number of coins was 140” or something similar. They really wanted to put coefficients in front of their variables, even when they had written down that their variables stood for the number of dimes and number of nickels! I’m not entirely sure how to instruct differently there besides maybe to do some more practice together.

 

You can download any of the pages I used for this unit here, most of them in both PDF and Publisher (editable) format.

Hack the System – Algebra 1

On Monday, I posted this tweet:

Tomorrow in Algebra 1 is either going to be awesome or a disaster…tune in to find out! #teach180 pic.twitter.com/I0VDJjdSXw

— Liz Mastalio (@MissMastalio) January 8, 2018

It was the culmination of several weeks of in secret stressing out at home and during prep periods to try to figure out how to make something awesome and fantastic happen – an Algebra 1 escape room!

I’ve done several escape rooms out in the real world – for the uninitiated, an escape room is basically what it sounds like: a room that you have to escape. In the publicly available ones, you sign up with a group, get locked in a room, and have an hour to find hidden clues, break codes, and solve riddles to unlock boxes, find more clues, and hopefully eventually find the room key to get you out!

In the education world, I had seen a few teachers creating these on their own, plus seen the officially packaged Breakout EDU kits. These kits are pretty expensive, so I had decided that I was going to create a breakout experience on my own just using locks and boxes I already had or that friends and family could provide me. I put a call out on facebook for extra locks or boxes that were lockable – pretty much everyone was like “wow, that sounds awesome but I don’t have anything like that” or “have you seen the Breakout EDU kits?” which yes, I had, but I don’t really have $150 to spare…and then the amazing Megan, one of our district lead teachers, commented and said “hey, the district owns some of the Breakout EDU kits that you can check out!” SCORE!

The Breakout EDU kits are pretty cool, and they even have pre-made escape scenarios for a few subject areas that you can download and then set up using the supplies in the kit. However, I am a perfectionist and I wanted the escape room to be exactly how I wanted it – I wanted LOTS of locks, with all of the combos only coming from solutions to math problems, and I wanted my entire classes to be solving problems to be able to escape. So, I ended up combining the Breakout EDU kit with a bunch of locks I purchased at Dollar Tree, and a few locks my mom had lying around, plus several other lockable containers and tricks that I came up with around my house.

So here we go, a breakdown of the breakout:

I split my classes into 5 teams. We have a small school and small classes, so in some of my classes this involved “teams” of a single student, and in some classes this involved partners. If you had bigger classes you may either need to come up with more teams or have bigger groups, and if you had bigger groups you would probably want to shorten the time limit. I gave my classes the entire class period – probably about 40 minutes once I gave all of the instructions and handed out supplies, and my 4th and 5th hours were able to escape right towards the end of class – close enough that THEY were nervous about not getting in the final box, but not quite close enough that /I/ was nervous about it.

The content in question is systems of linear equations. This activity acted as their review before their final for this term, so we had already learned all solution methods and practiced a bunch.

The first system on every team’s “Hack the System” sheet was set up to solve by graphing. They had to graph it on this crudely drawn map of our classroom that I made. The solution would lead them to the spot in the classroom where the set of puzzles they would need to solve was located.

Then, each team had 4 more systems to solve to help them decode their puzzles.

Team A

This team had these instructions before the 4th system: If the x-value of this solution matches the x-value of another solution, use the first 3 x-values to unlock the lock. If the y-value matches another, use those. If neither match, combine x and y values from each solution into a two digit number.

One of the x-values matched the x-value of their final solution, so the 3 x-values unlocked the green bike lock chain around the notebook.

On the first page of the notebook is written the combo to the lock on the small black box, which contains a key. This key unlocks one of the 6 locks on the final box.

Team B

I think this one was my stroke of genius. About a year ago, I shattered the screen on my iphone 5s. It was old enough that I just got a new phone rather than paying to fix the screen, but I kept the old one. Because I keep everything. And because I didn’t really know how to get rid of it. I packed up this phone with its shattered screen and moved it with me to my new house this summer. And as I was planning this activity, I remembered it! It still works, it just doesn’t have service anymore and the screen is shattered. So I wiped the memory, put a single note in the Notes app that was two cryptic words, and reset the passcode to be the solutions to this team’s set of 4 systems – xyxyxyxy. I set the lockscreen background so it says “unlock me and open Notes”. They opened the notes app and read the cryptic words which are the code to unlock the alpha-lock on the main box!

When my kids opened the iphone box to find out there was actually an iphone in it they were like “this is part of this? NO WAY! DO THE SOLUTIONS UNLOCK THE PASSCODE? THIS IS SO COOL!” Which made me really proud of this genius idea. You could make it work with any device that involves a password, really.

Team C

This team had an alumni cooler from my university (shoutout University of Northern Iowa Alumni Association!) The cooler has two pockets, both of which have double zippers – which means you can loop a lock through the two zippers and then it can’t be unzipped without unlocking!

This team had the same instructions before their final system as team A: If the x-value of this solution matches the x-value of another solution, use the first 3 x-values to unlock the lock. If the y-value matches another, use those. If neither match, combine x and y values from each solution into a two digit number.

It ended up that the final solution matched none of the other values, so they had to combine their values into two digit numbers (for example, (3,1) would become 31) that formed the combination. They had to just guess which lock on the cooler this opened. In that compartment, there was a seemingly blank piece of paper and a pen – but not just any pen…a pen with a blacklight on the end! The paper actually had the combination for the other lock written on it! When they unlocked that compartment, there was a key to one of the locks on the main box. Or, when a student in 4th period accidentally broke that lock when messing with it, I taped the combination to an extra lock I had inside that compartment.

Team D

This team had an actual lockbox that my dad had lying around from when he was the treasurer of my elementary school’s Parent Teacher Organization. It has a 4 digit combo lock on it from the Breakout EDU kit.

Before their final system, they had these instructions: Find the solution that’s x or y value matches that of this solution. Use BOTH values in that solution to unlock the lock, but if the x values matched, use ONLY x values from other solutions, and if the y values matched, use ONLY y values from other solutions.

The y value of that system matched that of the first system they solved, so the combo was x1 y1 y2 y3. Inside the lockbox was a ziploc bag with pieces of paper, and a makeup bag I owned with a double zipper that was locked by a combo lock. The pieces of paper are actually a puzzle, and the combo to that lock is written out in cursive words on the completed puzzle. Inside the makeup bag is a key to one of the main locks. The puzzle ended up being harder than I planned for it to be because it turns out that a lot of my students aren’t super comfortable reading cursive! So they didn’t know what the words said!

Team E

Team E was slightly different from the rest – I was out of containers that I could put a lock on, unless I wanted to bring my whole suitcase to school, which I really did not. So I decided that for the last two keys to the main box, I would hide them places in the classroom.

This team had these instructions before their final system: Discard the solution that has a matching x or y value with this one. The other two solutions correspond to map locations where keys are hidden – find them!

The x value of this system matched the x value of the other one, so the remaining two solutions had coordinates that they then plotted on our classroom map to give them a spot to search for the keys! I hid one of the keys in my classroom pencil cup, and the other in the holder for my SMART board pens.

This team’s setup would be a good way to add extra groups to your breakout activity – just hide extra keys!

If you needed to make the activity work for more students, I had the thought that some of the puzzles could be dead ends – lead to empty boxes, etc, or keys that don’t actually fit any of the locks. I really enjoyed the fact that every single group had to solve their systems in order to unlock the final box. Oh, and the final box had homemade chocolate chip cookies in it!

This took a LOT of planning to pull off, and I had to check and double check that I had written every system correctly to have the solutions that corresponded to the combinations. Some of these locks were reprogrammable, but some aren’t so I had to make it work with the combinations they already had. I was also glad that all of my students remembered how to unlock combination locks – our building doesn’t have lockers, so they don’t have to do it every day. If your building DOES have lockers, and there are spare lockers you could use and know the combos to, that could be a great place to put more clues!

It was really rewarding to watch my kids get so invested in opening that final box. I really loved this activity and I’m glad I put all the work in to make it happen! I really want to try to figure out a piece of content in Algebra 2 that will let that class do an escape room this year, because they were super sad when they came to class and saw all the lockboxes around the room and then got told that they weren’t for them 😦

Here are videos of my 4th and 5th periods getting the box open! Their excitement was so conagious!

Honestly this is some of the most fun I’ve had in a class period. Loved their enthusiasm and cooperation! #escaperoom pic.twitter.com/Dzy1qIIkL7

— Liz Mastalio (@MissMastalio) January 9, 2018

 

5th period escaped! I’m having so much fun today! pic.twitter.com/QJwgBb6uAw

— Liz Mastalio (@MissMastalio) January 9, 2018

 

I would share the activities I used for this room, but unfortunately they would be zero help to you since we don’t have the same locks! If you have questions about the way I set up the systems I can share some problems and solutions with you if you leave a comment or tweet me with your email address!

2018: Unashamed, Unafraid, Unfinished

I’ve decided I’m over resolutions. They don’t work for me – they make me feel bad about myself and have always felt like they’re made to be broken.

Last year, I came up with two phrases to guide 2017 for me:

prime number, prime life

action over inaction

Both of these have  been tremendously effective. Prime number, prime life comes out of the fact that 2,017 is a prime number, and just remembering that little fact throughout the year made me…happy. It made me remember that I could find some small thing in whatever situation that was good and to be proud of – to build my prime life.

Action over inaction made 2017 a year of constant motion for me. It made me take the options I would have normally passed over as being just too much effort. It’s easier to stay where you’re at. It’s easier to say you’ll wait until the summer, or next school year, or you’ll stop at McDonald’s just today and go grocery shopping tomorrow. This year, I took the action over inaction. I bought a house, started grad school, stopped drinking pop, started cooking way more for myself, cleaned more often, went and visited friends, called my mom more often. Living by this phrase WORKED for me.

 

So this year, I’ve come up with another one. Motivated by UN, meaning not. All the things I DON’T want to be in 2018. Or, looking at it another way, all the things I don’t want to hold me back.

 

UNASHAMED, UNAFRAID, UNFINISHED.

UNASHAMED – I don’t want to adjust the things I enjoy or am passionate about because of what other people are. This has always been a point of pride for me – I don’t watch a lot of the popular tv shows because I prefer reading, I love bands that “went out of style” years ago, etc. I also want to look at this word a different way in 2018 though. I want to be unashamed to call out someone’s racism, homophobia, or transphobia. I want to be unashamed that I didn’t think that rape joke was funny. I want to be proud to be a teacher and a single person and to make decisions that maybe you wouldn’t make. And yes, I still want to be proud of my interests and not think that “guilty pleasures” are a thing. I want to make my struggles public and feel good about them because they will get me where I’m trying to go, not to hide them all to try to feel like a perfect human!

I want to admit when I don’t know something, and not be ashamed of that because it is an opportunity to learn from someone else and to add more knowledge to my life!

UNAFRAID – I want to take risks, I want to speak out for those who can’t, I want to try new things and do things I never thought I could. I want to strive to reach things I dream of and not be scared of money or failure stopping me. I want to be the person who speaks up when something is wrong and have the hard conversations that I’m scared to have. I want to try new things and say “yes” when it’s easier and more comfortable to say “no”.

UNFINISHED – This word came from a professional development session our staff had before break, in which the speaker told us to “stay unfinished”. It was such a powerful sentiment – that we should never believe we are at the finish line of what we’re trying to do, because we can always become better, reach further, try harder. We should never stop learning and pursuing that knowledge that will help us to continue to become better and better and better, with no end! I want to read more diverse things, to try new things in my teaching and analyze what could be improved, I want to make my budgets better and become healthier and take care of myself more – I want to see all the growth I have made but also to see HOW FAR I CAN GO, which is ALWAYS further, because I am UNFINISHED.

One part of our school vision statement says that we will be “relentless in our pursuit to help all students overcome every obstacle to reach our potential”. I want to embody that phrase, but in my whole life. Relentless in the pursuit.

 

All of these UN words work together – Unashamed, Unafraid, Unfinished. All of them are about proudly being who I am and about working to improve that person to be even better than she was before. All of them are about speaking out and stopping the cycles of hate. All of them are about being in motion instead of being stagnant. And that is how I want my 2018 to look.