Book Recommendations (Vol. 05)

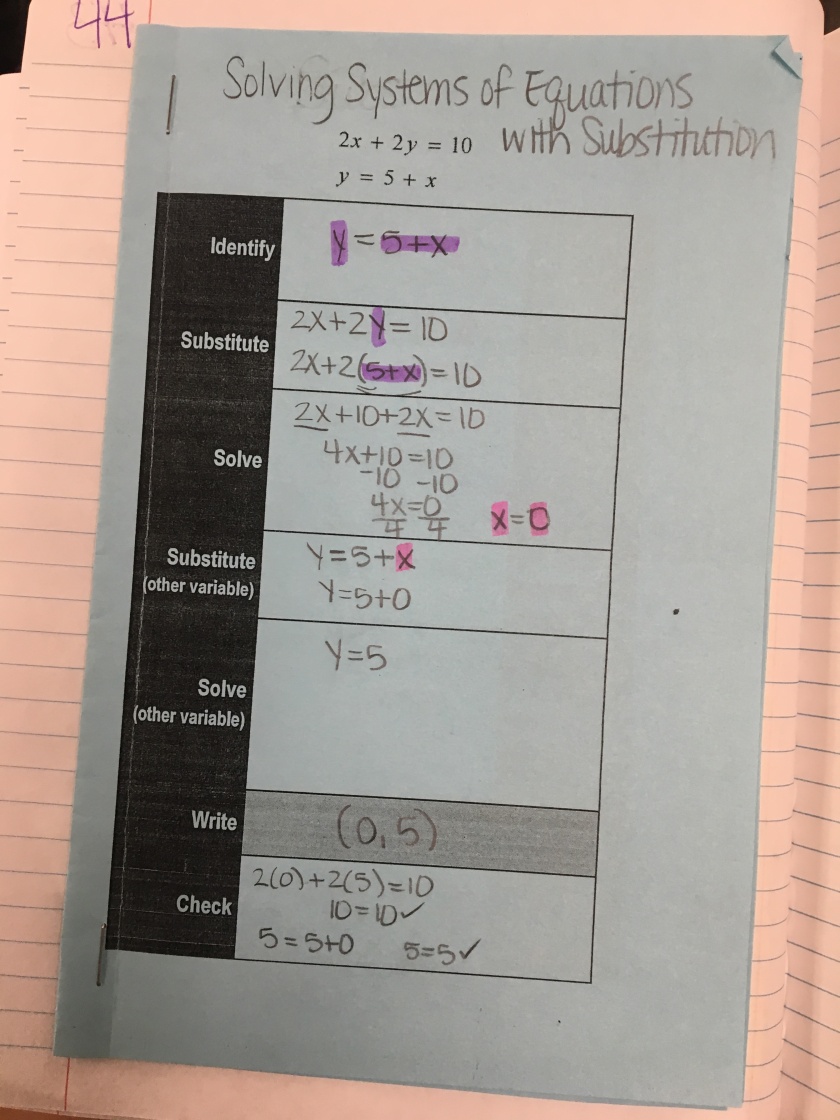
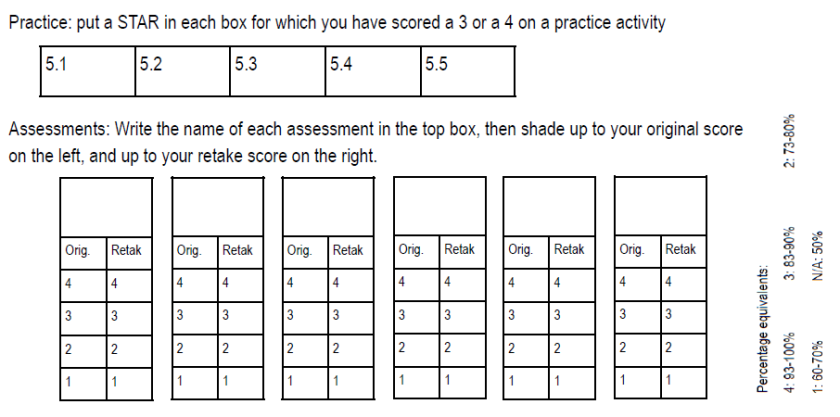
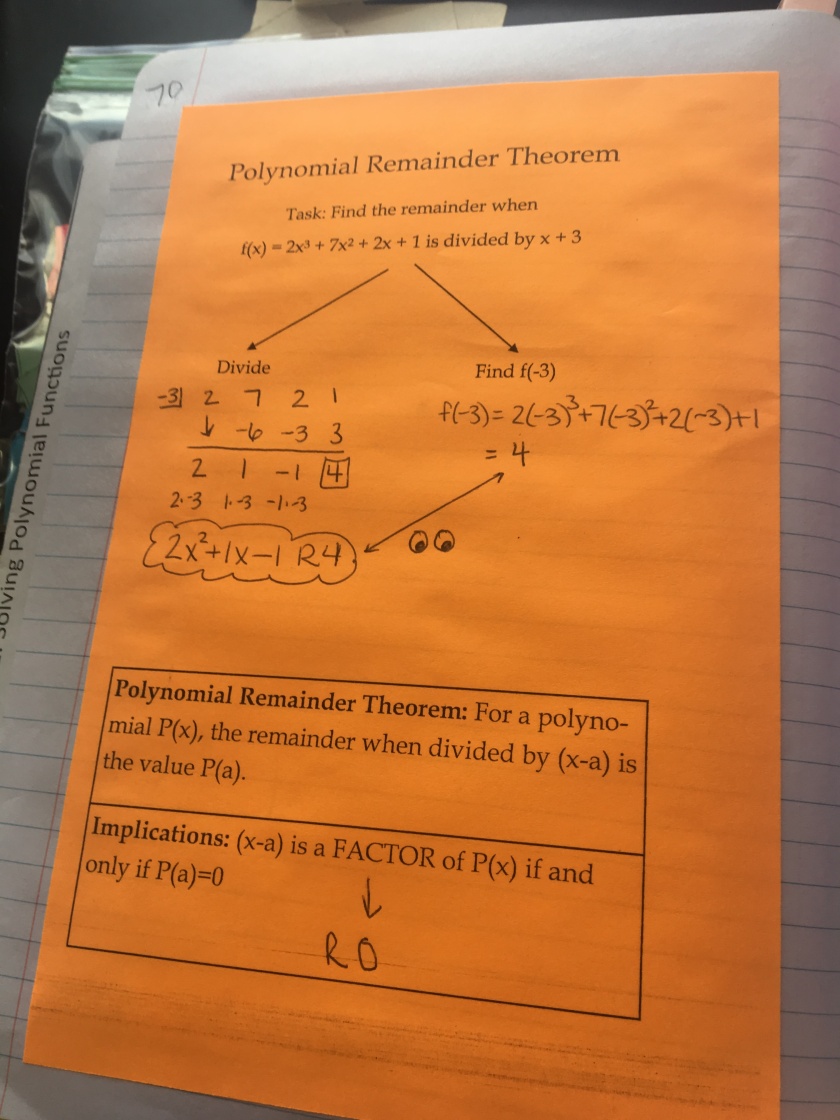
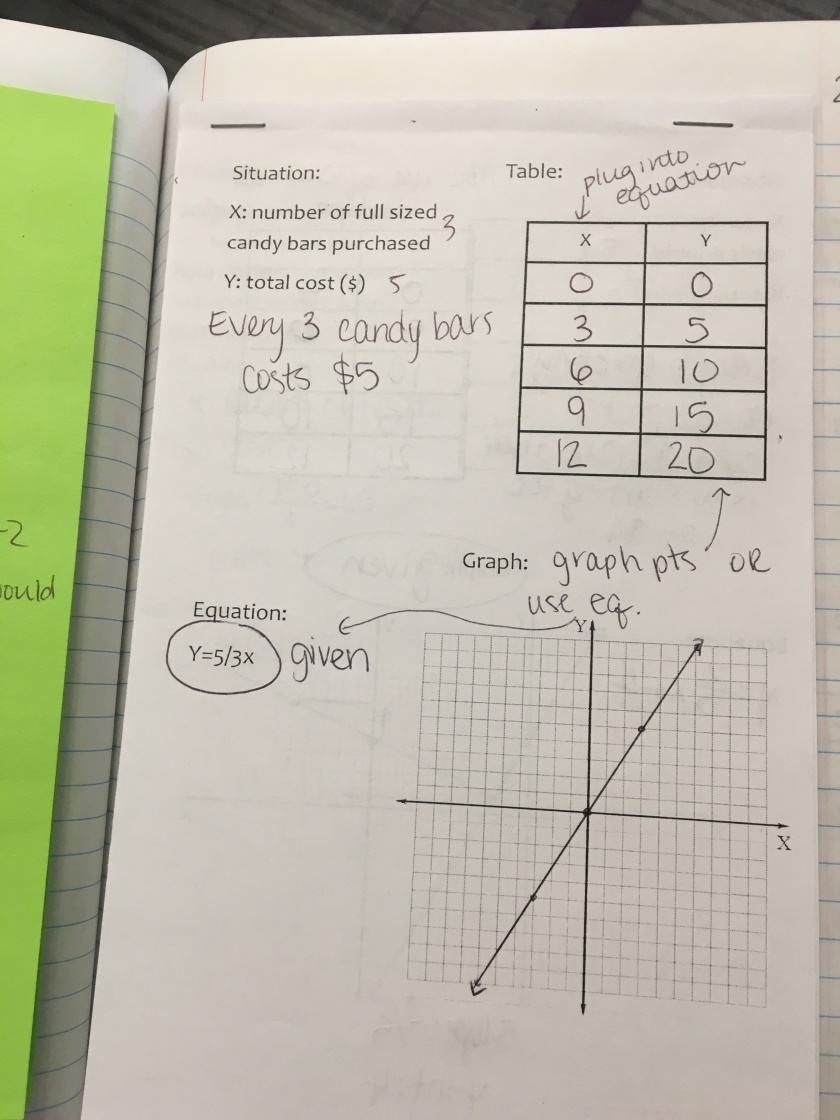
The first quarter of 2018 is almost over! What?!

That means it’s time for the next volume of my book recommendation posts. Each quarter, I post briefly about the top 5 books I’ve read in that quarter.

I’ve also recently started a mini project with Megan Morgan in my district to get teachers to share what they’re reading each Wednesday – you can follow the #dcsdpln hashtag or either of our twitter accounts to catch these posts! It’s super fun to see what my colleagues are reading, either for fun or for professional development opportunities, or for grad school.

Hey #dcsdpln! Time to show us what you’re reading over this spring break!! pic.twitter.com/23Ck9hbvyS

— Liz Mastalio (@MissMastalio) March 21, 2018

Anyways, on to my recommendations for this first part of 2018!

Read previous editions:

Vol. 01 | Vol. 02 | Vol. 03 | Vol. 04

I wrote my last post of 2017 when I had read 80 books. I ended up reading 88 books in 2017, and I have read 13 books in 2018 so far! Some of this top 5 is crossover from those last 8 books.

In Defense of the Princess: How Plastic Tiaras and Fairytale Dreams Can Inspire Smart, Strong Women – Jerramy Fine

This is an exploration of the attributes that make a princess – from our Disney heroes to the real life princesses still ruling across the world today. Fine explores character traits that they all have in common and how we can embody them in our daily lives, and explores her personal journey to becoming a princess.

This was a great (and fast) read – a defense of the idea that women can be strong, smart, and accomplished without giving up the desire to be feminine and to wear a tiara. It’s about how we can embrace the qualities that make a true princess – strength, kindness, diplomacy, serenity, rationality, and a giving spirit. I loved it. Perhaps a particularly relevant read as we all join in the fervor of welcoming a new princess, Meghan Markle!

Stamped from the Beginning: The Definitive History of Racist Ideas in America – Ibram Kendi

This book uses five major figures from American history to lend context to a comprehensive description of the development of racist ideas in our history. How did they begin, how did they spread, why are they still supported? Assimilationists, segregationists, antiracists, racists. Activists and artists. Every single historical figure you have heard of and many that you have not.

This is what it’s billed as – a comprehensive history of racism in our country. It was highly recommended by a professional development presenter that has spoken to our staff several times whom I intensely respect, and so I did not shy away from getting my hands on this 500 page work. I found myself pretty much every other page going, “I did not know that. I DID NOT KNOW THAT. Oh my god, I did not know that.” Lots of hard truths. Well worth my time reading the 500 pages. It’s not a light or fun or easy read, but if you want to see a better America I think reading this would be a good starting point on that journey.

Tempests and Slaughter – Tamora Pierce

This is the first book in a series following the mage Numair, first seen in the Immortals Quartet also by Pierce. We backtrack to when he was a boy, in mage school, meeting some of the other infamous characters from that quartet and discovering the roots of their relationships and powers. You see Numair (called Arram still here) discovering the vast potential of his power, his struggles with the idea of slavery and nobility, and the beginnings of some very dark goings on.

I grew up reading Pierce’s works in the Tortall universe – The Immortals, The Lioness, Protector of the Small, Daughter of the Lioness. I met her when I was probably 13 years old and have a signed book from her that is one of my most treasured books. Her works were a strong influence in developing my understanding of feminism and what it means to be a strong woman. With that in mind, the entire experience of reading this book I felt like I was coming home to Tortall (well, Carthak). Everything felt familiar and lovely and I was immediately enveloped in the Numair backstory. I am so deeply in love with this world and the other Tortall books formed so much of myself that I really couldn’t do anything but love this. It is very much a background building book for the rest of this series and someone not already in love with this world may complain that not enough happens in this one plot wise, but I cannot cannot cannot wait for more of this.

Truly Devious – Maureen Johnson

Stevie is obsessed with the Ellingham Academy mystery – many years ago, after the unique private school on top of a Vermont mountain opened, the founder’s wife and daughter went missing and are believed to have been murdered by someone using the name Truly Devious. Stevie gets accepted to the Academy with the goal of solving the case, but does not expect to get sucked into one of her own. Death is back at Ellingham Academy, and Truly Devious is, too. Maybe? Murder, or an accident? Related to the first, or a copycat?

I am genuinely atrocious at figuring out clues in mystery novels, and so I literally have NO IDEA what is going on with this case and cannot wait to get my hands on the next one to get some more information. This was gripping and mysterious from the start, and I love dual timeline novels as well. Who are these people Stevie is going to school with????? Is one of them a murderer???? What even? Maureen is an expert mystery builder, and all of her characters seem so incredibly real.

The Lost Colony (Artemis Fowl #5) – Eoin Colfer

10,000 years ago, after the battle for Irleand between human and fairy, all of the fairies moved underground. The demons, however, refused to surrender. Their warlocks used their magic to take the island they lived on out of time and into Limbo. Now, the spell is breaking down, and demons are starting to appear in the human world unexpectedly. Artemis discovers this, and so does another young human girl – could this be his intellectual equal? Of course Artemis and Holly have to try to figure out how to save the day.

The Artemis Fowl books are a series I began reading when I was in the preteen target audience, but only made it through book 3. I didn’t even realize there were more books until I saw an announcement about a film being made and looked into re-reading them. These books are still a delight to read as an adult – fairies, legends, technology, all of the dwarf potty humor – and I am almost finished with the series. This installment is my favorite so far because time travel always blows my mind to think about and it’s fascinating to explore the different structures authors use to set it up. The climax of this one had me on the edge of my seat, wondering how they would be able to get out of this situation because there are 4 more books so they have to survive! I highly recommend this series, especially if you are looking for books to suggest for your middle school aged kids, or one to read with your own kids or out loud in class….or just for you! There’s also a silly code that runs along the bottom edge of the pages in each book, which 10 year old me was STOKED to decode for herself but which adult me just looks up on the internet to read the translation 😉

 

Happy reading! Share your recommendations in the comments!

Algebra 1 Unit 3 Interactive Notebooks [Revised]: Systems of Equations

This year I’ve committed to posting each unit of both my Algebra 1 and Algebra 2 INBs.

My district is moving to a standards based curriculum, and has identified priority standards for every course. These are the standards we are required to address and assess our students over, so they pretty much form our units.

I normally teach year-long courses (47 minute classes per day). However, due to a lot of district specific things involving SPED students, a new course introduction, and graduation requirements, this year I taught a block course (94 minute classes per day) of Foundations of Algebra first semester, which covered skill gaps students would need to find success in Algebra 1. This semester, I am teaching (most of) those same students Algebra 1 on a block schedule. This means I get to start Algebra 1 from the beginning in the same year and revise my activities and INB pages!

You can find my Algebra 1 (year long class) INB posts here:

Unit 1 | Unit 2 | Unit 3 | Unit 4 | Unit 5

And my Algebra 2 INB posts here:

Unit 1 | Unit 2 | Unit 3 | Unit 4 | Unit 5 | Unit 6

And finally, my posts from this second round of Algebra 1 here:

Unit 1 | Unit 2

 

The third standard we cover in Algebra 1 is A.REI.6:

Solve systems of linear equations exactly and approximately (e.g., with graphs), focusing on pairs of linear equations in two variables.

I finally wrote a post about my journey through the other side of this index page – the evolution of my student proficiency log! You’ll notice if you’re a regular reader of my INB posts that I have been avoiding talking about them for awhile and that I only show the index side in these posts, and I am finally ready to share, but they deserve their own post 🙂

In my first post about this unit earlier this year, I said:

I pretty much like the way I organized this, except for the way I presented the elimination method

This means many of the pages I used are unchanged – but there are a few  besides just the elimination that I made some minor changes to. However, I probably won’t have as much to say about each skill and you can read my original post about this unit to get more insight into the other pages.

Skill 1: I can determine if a given point is a solution to a system of equations

 

Getting down those definitions and learning how to check possible solutions. None of my students use the “storing values in the calculator” method to check their solutions, but our curriculum coordinators in the district specifically told us that we need to show them this, so it’s there.

Skill 2: I can graph a system of equations to find the solution

First we talk about the possible outcomes when solving a system of equations. This was more fluid for these students than my first Algebra 1 group this year, because I added a page that is essentially just like this into their original solving equations unit. The only different thing about solving equations really is that you also have to know what the graphs look like, and that there is an x AND y value.

I changed the graphing pages to be cleaner and reduced the number of examples. Really, I have discovered that my students just need more practice graphing – by themselves, without me. So these notes have one example of each possible outcome, and then they practiced. I like these notes, but I really struggled to get the x and y axis to show up in Publisher the way I wanted to, so I ended up just drawing them in with a permanent marker before I made copies. I then tried to scan this copy so that I could just print it in the future, but the office copier hates me recently and it did not turn out well. It’s included in the files anyway, so you can see how poorly this ended up. Drawing in the axes each time you make copies is really not that bad, compared to a long and drawn out fight with the scanner!

Skill 3: I can solve a system of equations when given the value of one variable

 

The only thing I changed here from before is that we still did a notice/wonder with the systems included in these notes, but I did not have them attach that notice/wonder to their notes, we just did it on the board. I still really like this as an intermediary step, since it’s essentially a review of solving equations once they substitute in that known variable.

Skill 4: I can solve a system of equations using substitution

I kept this one exactly the same. Upon using it with this group of students, I think it may be beneficial to cut the number of examples here as well, like I did with the graphing. We hand wrote an example with no solutions in the center fold of this stapled notes section, since there ends up being two blank half pages in the middle, but I plan to just change it to be two one solution examples and one special case example.

Skill 5: I can solve a system of equations using elimination

I am moderately happy with how I set up the elimination method this time. We started with looking at what happens when you multiply entire equations by a constant and discussing what elimination means as a word. We looked at the equation x+2=4 and solved it, and then I asked what would happen if we multiplied the entire equation by a number of their choice. They chose 4. They were adamant during the entire process that the solution was now going to be x=8, even down to the moment where we were ready to divide 8 by 4. Their minds were blown. I think they’re still mad at me about it. But it stuck in their heads that multiplying these equations by some constant doesn’t affect their answers!

We then began with systems that are already set up for elimination – where either the x coefficients or y coefficients are already opposites. This was the step I missed last time. This was easy for my students.

Then we moved into how you can GENERATE this opposite effect if it isn’t there to begin with. I think one of the issues is that my students just aren’t fluent enough in multiples to think through this well. “What do 8 and 12 both go into?” is the hardest question in the world for them. I think I need to bring multiplication tables into this the next time I teach it. Anyways, we practiced just getting these opposites a few times, and then went into the last page of notes which is three examples of completing the elimination method all the way through to a solution.

I think part of my issue with teaching elimination is that it is my personal preference for a solution method when I solve systems myself, and so I do it so automatically that I think I have trouble thinking through all of the intricacies that my students are going to struggle with. Would love to accept suggestions from people on this one!

Skill 6: I can write and solve a system of equations to represent a situation

I didn’t change these last two pages at all from the last time I taught this. I did, however, struggle to get students to actually consider the most efficient method when they were solving systems. I tell them that they can choose whatever method they want to use, but I kind of screwed myself over with that statement when several of my students decided they were going to solve every single system by graphing it – and they were not quite proficient in graphing. I ended up encouraging them to look back at these notes and having them think about what they system was “set up for” and I won some of them over. The main issue was that they wanted to use graphing every time (or some of them were on team substitution or team elimination), but they really only knew how to use that method if the system was already set up for it. So they would go, “I want to graph this system but what is the y intercept” and I would respond “that equation is not in slope intercept form” and then they would give up. I’ll have to work on that.

 

You can find the files for these pages here, including a subfolder with the previous versions of the pages.

 

 

 

Student Proficiency Logs: A Journey

If you have been following my Interactive Notebook posts closely, you will have caught a hint of this journey that I’ve been on. It really begins last year, before I was using INBs in all of my classes, and it did not involve INBs at the time.

One of my big goals even in my first year teaching was to try to help my students process where they are at in the course. I want them to be able to answer questions like:

1. How did I do on this assignment?
2. What does the score I got mean?
3. What skills do I need more work on?
4. What am I missing that I need to make up?
5. What do I understand well / what am I proficient at?
6. Do I need to schedule myself for Miss Mastalio during intervention period?
7. What is the end goal of what we’re doing in class?
8. What are we actually working on in class right now?

My first two years teaching, I printed out student grade reports at the start of each week, passed them out, and answered any questions students had about them. That pretty much only answers questions 1 and 4 on the list above. (Maybe question 6 but we did not have an intervention period then so it didn’t apply.)

During the 2016-2017 and 2017-2018 school years, I created a grade log form to go with this Monday activity. I still printed out grade reports for each student and handed them out on Mondays, but alongside this log page.

In addition to questions 1 and 4, this now started to address question 5, and 6 came into play as we added a form of our intervention period.

This year, our district is starting the transition to standards based grading. With this change, I have thought a lot more about the meaning of scores and how to effectively communicate goals and proficiency to students.

The past several years, I also had students filling out an opener sheet daily. They were tasked with copying down the opener problem and the lesson objective for the day, 4 out of 5 days every week. This kind of addressed question 8 from the list.

This year, I changed my grading policies so that student practice work does not affect their final grade anymore – only assessment materials do. I knew that this meant it would be hard to convince them of the usefulness of writing down openers and objectives, so I decided to do away with that sheet. We still do an opener and read the objective daily, but we just do them as a class and don’t record them individually. That’s another story as well (perhaps another blog post?) but I think it’s been pretty equal in effectiveness to what I was doing before, plus I don’t have to grade 60 opener sheets every Friday.

I also started using INBs with all of my classes this year, so my thought was that I could incorporate the grade log and the opener sheet components into something in their INB. The start of this school year got away from me, so I didn’t quite get this done. My index pages for my INBs do address questions 7 and 8 though – they clearly show all the skills that we are working on day to day, and also describe the big standard that is the end goal of each unit. We talk through how these all fit together each time we add to our INBs. The objectives I post in my classroom are exactly the same wording as our skills in our notebooks. This part of my question list started to fit together nicely.

On the back page of the indexes, where it wrapped around the other side of a page in the notebook, I originally just had a large heading with the name of the unit. I realized that something similar to my old grade logs could go in that space, and began to work on this proficiency tracking log.

Wow, is this way too complicated or what? Can any of you even figure out how to fill it out? What was I thinking??????

The thought was that students would check their grade on a chromebook or on their phone (we are being limited more severely on printing/copies this year so I no longer feel like I can print a grade report for each student each week) and then fill this out with the results. At this point, we’re really getting to all the questions on my list. They know that scores of 3 or 4 are proficient, and 1 and 2 are not. A 0 means they are missing that assignment/assessment. Question 1,2, and 4, check. Questions 3 and 5 are answered by them identifying which skills they are proficient in. Question 6 can be answered by them considering their grade and identifying if there’s an assessment they’re missing. Questions 7 and 8 are answered by the index side of this page and our discussions in class.

But this form is just way too much for a quick check and is harder to figure out than it is helpful. I immediately realized this when I tried to have students fill it out for the first time. It was bad.

 

Back to the drawing board!

I’ve finally come across one I like:

They check Campus and their grades, and then use those scores plus their own reflection on their learning to give themselves a proficiency mark from 0-4. I have signs posted in my room that describe how each of those numbers would feel, and I give them a brief reminder on Tuesdays when we mark this: “0 means you literally have no idea at all what the skill even is, 1 is you just starting, 4 is give me any test right now with this and I will ace it”

I write on the board which skills they should be marking – the ones we’ve covered in the last week – and remind them that they can adjust old markings if they feel they’ve improved.

Questions 1,2, and 4 are addressed by their quick check of the gradebook. Questions 3 and 5 are addressed by their reflection on the skill statements and their scores. Question 6 is easy if they missed an assignment, or if they seem to be marking all 1’s and 2’s I suggest they schedule themselves for me during intervention time. Questions 7 and 8 are essentially the questions they should be answering to themselves as they reflect.

I’m happy with this. I feel like it could benefit from some written record of their scores, but I don’t want to veer into making it too complicated again. I may add some boxes in the empty space at the bottom for them to record assessment scores for the unit. The goal is for this to be a quick and automatic process at the start of class each Tuesday, and I think we’re getting closer to that.

I also like the way this looks because I think it’s easy to explain to a parent, and so I could use this at conferences to communicate strengths and weaknesses of each student without relying so heavily on the student’s grade for that explanation.

 

Do you use any sort of grade/proficiency tracking with your students? How do you run it and do you like it? Leave a comment and let me know, I’m definitely looking for better ideas here!

You can download an example of this index/proficiency log page here for PDF or here for Publisher.

Algebra 2 Unit 6 Interactive Notebook: Solving Polynomial Functions

This year I’ve committed to posting each unit of both my Algebra 1 and Algebra 2 INBs.

My district is moving to a standards based curriculum, and has identified priority standards for every course. These are the standards we are required to address and assess our students over, so they pretty much form our units.

You can find my Algebra 1 (year long class) INB posts here:

Unit 1 | Unit 2 | Unit 3 | Unit 4 | Unit 5

And my Algebra 2 INB posts here:

Unit 1 | Unit 2 | Unit 3 | Unit 4 | Unit 5

And finally, my posts from a 2nd go around I’m teaching of Algebra 1 here:

Unit 1 | Unit 2

Our 6th prioritized standard in Algebra 2 is A.APR.2:

Know and apply the Remainder Theorem: For a polynomial p(x) and a number a, the remainder on division by x – a is p(a), so p(a) = 0 if and only if (x – a) is a factor of p(x).

Really, the goal is to use the remainder theorem to solve polynomial functions.

I am extremely happy with the way this unit turned out – I think the sequencing was good, I think the notebook pages I made are effective, I think we did good activities along the way, and of course the most important, my students demonstrated really strong proficiency on this standard. I think it’s my best-taught unit so far this year, so I’m taking a moment to acknowledge myself.

Skill 1: I can multiply polynomials

This is a review – we touched on multiplying in their second unit when they worked on quadratics, but that was around October and I don’t expect them to automatically remember it in February. I also gave them two different methods to use – the distributive property and the box method. The instructions for the box method one come from Sarah Carter, but I didn’t keep all of the same examples. Every single one of my students used the box method after we did these notes, but I think it’s worth them knowing that that isn’t THE ONLY WAY to multiply polynomials. We ended up practicing this skill on a day we had a late start from snow (and we watched Olympic highlight videos for the first 20 minutes of class…) but they still got enough practice to feel confident about it again.

Skill 2: I can divide polynomials

We learned three different methods to divide. We did the same examples with all three methods, so that they could start to see the relationships between the methods. I then let my students choose their preferred method, with the caveat that synthetic division only works in certain cases. Pretty much all of them settled on synthetic division when it works and the box method when it didn’t. I think they had traumatic memories from long division with integers that made them not want to do long division with polynomials. We learned a lot of vocabulary that they did not remember, also: dividend, divisor, vinculum….

I need to add some more words to the box method notes because later, my students kept forgetting where the dividend showed up – did it go on top or in the boxes? A sentence or two in their notes would help with this.

Skill 3: I can use the Remainder Theorem to identify factors and zeros of polynomials

Before we did these notes, we did an exploration where my students basically figured out what the Remainder Theorem was on their own. True, the statement of it is what the standard says so it was already on their index page, but the way it is stated in the standard is extremely mathy and not in student friendly language.

Once they figured it out, we put it into notes with this one example. We used this to practice identifying values of f(x) and to find factors.

Skill 4: I can solve a polynomial function

I broke this down further into several pieces – we started with just factoring the polynomials. This began with a piece of reasoning about how the Remainder Theorem would be useful to us that made my students incredibly frustrated because I would not tell them the answer until they figured it out themselves.

Why is the Polynomial Remainder Theorem useful? and using it to help us factor polynomials! #teach180 pic.twitter.com/4dHehB3RMj

— Liz Mastalio (@MissMastalio) February 23, 2018

We had already figured out that if we could find factors, that would be nice, because they go into the polynomial evenly without those pesky remainders! So I wrote the stuff in black up on the SMART board and posed the question, “where on the graph would I find P(a)=0?” They were stumped. We had to break it down. What does it mean that P(a)=0? We eventually figured out that we were looking for where y=0, and then we were stumped for a bit longer, and then we figured out that we needed x values, and eventually one of my students yelled out X-INTERCEPTS! and they were all very excited.

Then I handed them the factoring notes page and they were like, “Miss Mastalio, it’s right on here, why couldn’t you just give us this???” Oops, made them discover math on their own. Anyways, once we figured out we needed the x-intercepts, it was pretty straightforward from there. They graphed, looked for integer x-intercepts, and then used synthetic division. I actually had the realization for the first time ever that you don’t even need to have them look for a GCF first – if you use the synthetic division, you’ll end up with the GCF as a result at the end! UNLESS there is an irreducible quadratic in the factorization, but I decided I was ok with not having a GCF factored out in that circumstance since our priority standard is focused more on using the Remainder Theorem to find factors and our assessment is mostly focused on using this to solve the polynomials, and you’ll still end up with the same solutions whether or not you factor out the GCF.

ANYWAYS, we factored polynomials to an end result of only linear and quadratic factors. Then, we reviewed solving quadratics with complex solutions. This is one of the units that I was not entirely happy with when I taught it at the start of the year. I did not focus enough on the complex solutions and focused more on solving quadratics with real solutions, which is an Algebra 1 standard. When I change this next year, I may not have to do as intense of a review during this unit. We used the Quadratic Formula until we hit the “taking the square root” point of simplification, and then we looked separately at simplifying those square roots. I like the way I explained this at this point in the year, and with a few adjustments to make it an introduction instead of a review, I think I will use these notes in our Unit 2 next year to teach it the first time.

Once we had practiced factoring and finding those quadratic solutions, we finally focused in on solving. I broke it into 4 steps: factor, find the linear solutions, find the quadratic solutions, write your solutions as a list (making sure to check that you have the same number of solutions as the degree of the polynomial). The students responded really well to writing the synthetic division as a continuous chain, once they realized that they needed to divide their answer by the next factor and that they could get away with not rewriting it.

 

You can find these files here, in PDF and editable forms!

Algebra 1 Unit 5 Interactive Notebooks: Exponents and Radicals

This year I’ve committed to posting each unit of both my Algebra 1 and Algebra 2 INBs.

My district is moving to a standards based curriculum, and has identified priority standards for every course. These are the standards we are required to address and assess our students over, so they pretty much form our units.

You can find my Algebra 1 (year long class) INB posts here:

Unit 1 | Unit 2 | Unit 3 | Unit 4

And my Algebra 2 INB posts here:

Unit 1 | Unit 2 | Unit 3 | Unit 4

And finally, my posts from a 2nd go around I’m teaching of Algebra 1 here:

Unit 1 | Unit 2

Our 5th Algebra 1 Unit focuses on the N.RN.2 standard:

Rewrite expressions involving radicals and rational exponents using the properties of exponents.

This standard assumes that students are already proficient in the exponent properties using whole numbers, but that is certainly not the case for my students. Most of them act like they have never seen the exponent properties before, and some of them don’t even know the definition of an exponent. So we start at the beginning – here is the skill breakdown I used.

Skill 1: I can combine exponents with like bases

In retrospect, I should have really focused this skill in on a single property at a time, very simple problems only. My students were starting with so little understanding of this that they became overwhelmed as soon as we got to the inside of the example booklet where they had to use more than one property in the same problem. When I teach this next, I plan to do a lot more examples here with one isolated property in them, and then move into combining problems with a few examples and in the scaffolded practice activity I created.

However, I do like teaching the properties as one generalization involving the order of operations instead of teaching them as several separate properties. We start this unit off with an exponent exploration that helps the students discover these patterns and properties, so formalizing them in one cohesive swoop is nice and gives them less to remember, plus they always have the expanded form strategy to fall back on if they forget.

Skill 2: I can rewrite negative and zero exponents

I am really happy with the front page of this and all the pattern finding we did while we filled it out. Once my students found the dividing by 5 pattern, they immediately found decimals for the negative exponents, and I asked “how else can you write decimals?” “Fractions…” they replied with a groan in every class, but then all of them persevered in coming up with fraction equivalents for 0.2, 0.04, and 0.008 – either from things they knew or by using place value and reducing the fractions. We wrote the “rules” for negative exponents and zero exponents only after we figured out what was happening, and I really think this developed a strong understanding.

Many of my students struggled with not knowing how we moved from step to step in the examples when they were looking back on their notes, so I think next time we will bust out the highlighters and highlight the portion of the problem we are addressing in each step so that it’s more clear when they look back on their notes.

Skill 3: I can rewrite exponential expressions in equivalent forms

I love this “goals when ‘simplifying’ exponent expressions” checklist. When are you done simplifying? How do I know that this is the “simplest”, since that word is subjective? This tells my students what I am looking for when I am grading and makes the process of “simplifying” much more objective.

Then we took the opportunity to practice some of the “really long” problems, as my students say.

Skill 4: I can convert between exponential and radical forms

I like these notes, but I need to include more that involve variables in the examples and less of the “convert and evaluate” examples. Perhaps reverse the ratio of these types. Fun part: my students are now all horrified that imaginary numbers exist and that they’re eventually going to have to do something with them. That was a really awesome portion of the lesson, actually, to just watch their faces as their minds exploded a little. One of them, when he put the expression into his calculator, informed me that he got an error message. I responded “YES! What does it say?” and he just went “Nooooo. I already don’t like this.”

Skill 5: I can rewrite radicals in equivalent forms

I need better instructions for the Sieve of Erasthothenes. Somehow. I looked at Shaun Carter’s activity and perhaps that is a better way to go for next time. Half of my students have an incorrect list of primes in their notebooks because they did not follow my instructions. For many of them, the problem was as simple as counting by threes incorrectly though, and I am not really sure how to fix that. I do think it’s beneficial to do this and not just GIVE them a list of primes, but it’s not helpful if they end up with the wrong information. I’ll keep thinking about this one.

I love teaching the two methods of prime factorization and letting the students choose which one they prefer. It varies drastically student by student and they are all incredibly defensive about their preferred method, which I find hilarious.

I got the prime number coloring chart and the simplifying radicals instructions from Sarah Carter, although I changed the examples on the inside. We played a really cool game to practice this that the students really found useful – inspired by this post from Mrs. Awadalla, resulting in this activity by me.

Doing an Algebra 2 activity that involves drawing cards – as a result, I’ve taught several students to shuffle today! pic.twitter.com/dShIDhf2i5

— Liz Mastalio (@MissMastalio) March 1, 2018

 

Files from this post that I created can be found here, in PDF and editable forms. If I got the resource from another teacher, the place you can find it is linked within the post 🙂

Algebra 1 Unit 2 Interactive Notebooks [Revised]: Writing and Graphing Linear Equations

This year I’ve committed to posting each unit of both my Algebra 1 and Algebra 2 INBs.

My district is moving to a standards based curriculum, and has identified priority standards for every course. These are the standards we are required to address and assess our students over, so they pretty much form our units.

I normally teach year-long courses (47 minute classes per day). However, due to a lot of district specific things involving SPED students, a new course introduction, and graduation requirements, this year I taught a block course (94 minute classes per day) of Foundations of Algebra first semester, which covered skill gaps students would need to find success in Algebra 1. This semester, I am teaching (most of) those same students Algebra 1 on a block schedule. This means I get to start Algebra 1 from the beginning in the same year and revise my activities and INB pages!

I took a poll on twitter and many of you said you would like me to still include pages that I didn’t change, so that’s what I’m doing, but I’m not going to write extensively about those pages.

 

You can find my Algebra 1 (year long class) INB posts here:

Unit 1 | Unit 2 | Unit 3 | Unit 4

And my Algebra 2 INB posts here:

Unit 1 | Unit 2 | Unit 3 | Unit 4

And finally, my other posts from this 2nd go around of Algebra 1 here:

Unit 1

 

Our second unit in Algebra 1 addresses A.CED.2 for linear functions only:

Create equations in two or more variables to represent relationships between quantities; graph equations on coordinate axes with labels and scales.

If you read my INB post from my first time teaching this standard this year, you will quickly realize that I did NOT LIKE how I organized it or presented it. I was excited for this opportunity to change a lot of the pages and see if I got better results. I think this is better, but I’m still putting this down as my weak point in Algebra 1 instruction.

Skill 1: I can determine if a relationship is a function and if it is linear.

I did not change the definition page or the function machine page from last time, but I did add a bit of organization to my “how do I tell if a relationship is linear?” page. Students glued examples and non examples from Sarah Carter’s Function Auction under the Frayer model after we did the Auction and discussed our results, instead of drawing examples and non examples like you see in the picture. Partners split the Function Auction sheet between them, and each student chose 3 examples and 3 non examples to glue in.

Shortly after teaching this skill, I had to do a grad school project that involved reading and reporting a TON of research on teacher and student image of function, so I already have tons of things that I’ve been thinking about that I would like to change about this next time. Mainly, that I want to spend way more time on this idea and really cement it in, and not treat it as much like an “intro” to the rest of the unit.

Skill 2: I can find the slope, y-intercept, and x-intercept of a linear function.

I broke slope down into representations: tables, graphs, and situations. We get to equations later. Of course we watched Slope Dude! The other page addresses intercepts, and I like the way it turned out. The definition boxes on the front come from Sarah Carter, and I just combined them with examples on the inside.

Skill 3: I can identify and evaluate all forms of a linear equation

I love that I made this a separate skill this time. Skills 1-3 would be what I was thinking of when I said I would have an “intro” part of the unit in my last post, although I didn’t end up actually separating it into two units. My students did a much better job at recognizing slope-intercept, standard, and point-slope form this time. I would like to add another page here about converting between forms, because although we discussed how to find slope and a point to use to graph directly from each form, my students still struggled a lot with graphing when the equation is in standard form. They were also incredibly thrown off with the few equations that they encountered that weren’t quite in any of these forms.

Skill 4: I can graph all forms of a linear function

I made two nearly identical pages here – one for creating a table of values and graphing, and one for identifying information from the equation like slope and y-intercept and graphing that way. All of my students except one abandoned making the tables after we learned the other way, but I think it was worth it to still discuss. We busted out my giant graph made from a bedsheet, which was really good because it turns out that my students really struggled with graphing ordered pairs, so it was extremely good practice to give each of them a checker and make them graph one of the points from our table!

I almost want to make a THIRD page that is similar to these two that shows how you can get every equation into slope-intercept form and graph that way. Anyone else think that would be helpful? Leave a comment 🙂

Skill 5: I can write an equation for a linear function

Here you can see my intense scaffolding, especially when you compare it to similar skill based pages from the first time I taught this skill this year. My students in my year long Algebra 1 got so incredibly overwhelmed by all the steps (define variables, choose a form of equation, find the slope and intercept, write the actual equation), that I decided to make EACH STEP a separate page in our notebooks and we also practiced each step separately! If you look at these pages closely, you will see that I used the exact same word problems throughout each step of the process. In particular, my students got really annoyed at Ben and his walking/running, and Conner and his ridiculous bank account that we are all jealous of after looking at these same situations for about 4 straight days of class!

When defining variables, I ended up changing the prompt sentence from _____________ depends on ______________ to “If I change ___________________, _______________ changes because of it” because my students were really struggling with the word “depends” – they could not reliably tell me if the sentence “cost depends on number of sweatshirts ordered” or “number of sweatshirts ordered depends on cost” made more sense. The change seemed to be effective for them.

I really liked this decision and I think it really increased my students’ proficiency in writing equations from word problems.

Skill 6: I can generate all other representations of a linear function when given one representation.

This is always going to be a rough skill, I think. These days of class inevitably contain endless complaints of “this is too much!” and refusing to write, etc. I don’t know of any way to make it seem like less work to the students, because the whole point is that you have to be able to create ALL of the representations. So you have to make them all. I did cut the number of examples in their notes down a bit from last go around though (I took one of the examples from this file out after I printed them) and was more explicit about identifying which representation you were given to start with. I think this skill is a good exercise in thinking systematically, which is a weakness for most of my students. In the end, this strengthens all of their other skills from this standard and I’m going to keep including it, even through all the complaints.

 

You can download the files for the notebook pages I created myself here, in PDF and Publisher/editable form, or find links within the post to pages I got from other teachers.

First Dance

My school hosted its first ever dance last night. We’re an alternative high school, so we are laser focused on getting these students to graduation – we don’t have extra curricular activities like sports, and the few clubs we have meet during school hours in our intervention periods.

Our students can go to prom or homecoming at their assigned home high schools, but they decided they wanted a dance of their own. A dance that was uniquely us (and we are UNIQUE).

I learned a lot from this dance

People donated dresses to us so that our girls who couldn’t afford to go buy one could still feel fancy and beautiful. Volunteer hair stylists and makeup artists came in beforehand to help in this process as well.

Watching these girls get so excited about their dresses and their hair and asking for a bajillion pictures was so sweet to watch and made me think of how much I take the opportunities I have to dress up and feel especially pretty for granted. This was for most of them the first time they had gone to any sort of fancy event, and you could tell how special and beautiful they each felt.

At the dance itself, I watched my students being totally themselves – laughing and dancing with their friends. Students who came alone were brought into groups of friends dancing – no one was alone at this dance unless they wanted to be. One of the things I love most about our building is this – our students really take to heart the inclusion of every student. Everyone fits somewhere.

The interactions between students and staff during the dance were hilarious to watch, but also important I think. Them seeing us dance, together and with them, being silly and outside the classroom environment, probably changed a few Student/staff relationships. I’m sure our very uncool dance moves made many a Snapchat story last night. I know I embarrassed many students with an interpretive dance to the slow songs.

All in all, it was plain good fun. I think we all could benefit from the chance to just have fun with our students – no assignments, no grades, an even playing field of fancy dresses and ties and questionable dance moves. A chance for everyone to laugh at each other and feel special and included and part of something.

We got to celebrate being Mavericks last night, and it was lovely.